Keywords
Old Babylonian, mathematics, economics
§1. General presentation[1]
§1.1. Tablet YBC 4698 provides an interesting overview of the mathematical approach to economic problems in the Old Babylonian period. Although well preserved (see the photo posted at https://cdli.mpiwg-berlin.mpg.de/P255010), the text has been poorly published. In MKT 1, Neugebauer confessed that he was not able to publish the text.[2] In MKT 3, he made little progress. He transliterated the text leaving many signs unidentified, and translated only a small portion of it (MKT 3, 42-45). Neugebauer recognized that his difficulties came mainly from the fact that he was not familiar with economic terminology.[3] Thureau-Dangin improved the reading of some words, and provided a transcription and translation of section 16, the rest of the text remaining quite obscure (Thureau-Dangin 1937b, 89-90). In TMB, he only quoted the text (p. 214) and referred to MKT 3 and to his previous paper.[4] Friberg, in different chapters of his 2005 monograph (2005: 23 n. 10, 60-61, 67-68, and 215-218), made substantive improvements to YBC 4698’s reading and translations, as well as interpretation of problems 3, 6, 7, 8, 9 and 10. However, he considered the problems as isolated entities. In this article, we try to look at the text as a whole, and to understand how the different problems are interconnected.
§1.2. Few mathematical texts dealing with economic matters are known to date. As noted by Neugebauer and Sachs (1945: 106), “the published mathematical texts concerning prices are badly preserved or obscure for other reasons.” Among these economic mathematical texts are VAT 7530, MLC 1842,[5] as well as some texts discovered after the publication of MKT and MCT, such as texts from Susa (TMS 13 and 22[6][), from the Diyala Valley (IM 54464),[7] and from southern Mesopotamia (MS 3895).[8] Nowadays, the reading and interpretation of YBC 4698 can be improved as we profit from a larger comparative material, from a better understanding of economic texts and mathematical series texts, and from Friberg’s work on “combined market rate exercises” (Friberg 2007: 157-168). Thus, in this article we can provide an almost complete transliteration, translation and possible interpretations of the preserved portions of the text.
§1.3. The type of tablet is M(2,2) (multi-column tablet, with 2 columns on the obverse and 2 columns on the reverse). The text contains 17 problem statements on interest rates, prices and profit, and ends with a colophon which indicates that the tablet is the third of a series (dub 3-kam-ma). Thus, YBC 4698 belongs to the small collection of 20 mathematical series tablets known to date. The origin of this tablet is unknown. However, the series tablets probably date from the end of the Old Babylonian period (late 18th century or early 17th), and may come from central Mesopotamia (perhaps Sippar or Kish). (See discussion on date and provenience of mathematical series tablets and related bibliography in Proust 2009b: 169-170.)
§1.4. Although damaged, one guesses that the colophon contained the number of sections (17 im-šu) as well, though this reference seems to have been erased in antiquity. Each of the 17 sections contains the statement of a problem, without any indication about the procedure of resolution. All of the statements deal with the same general topic, namely economic issues. The writing uses mainly sumerograms, but it is not clear if the text is written in Akkadian, in Sumerian, or in a completely artificial language (see discussion in Proust 2009b: 170-171).
§1.5 The list of 17 problem statements found in YBC 4698 can be divided into several homogeneous groups (for more details, see §6):
#1-2: The interest is given, find the principal.
#3-5: The rate in-kind or values of two kinds of goods are different; find the price of each of them in various conditions.
#6-11: The purchase price and the selling price of a good are given; find the profit (and reciprocal problems).
#12-14: similar to #3-5, but the resolution of the problem involves division by non-regular numbers.
#15-16: Different ratios are used to evaluate goods. Find the values or rates.
#17: Rates are used to evaluate value of two kinds of stones. Find the weight of the stones.
§2. Terminology
A glossary is provided in §8. Here, we emphasize the technical meaning of expressions used in economic problems.
§2.1. The suffix –ta. As an economic mathematical text, YBC 4698 exhibits some features and terminology similar to those found in economic archives. For instance, the ablative suffix –ta is used to indicate rate per unit. The same layout is described by Snell (1982, 39) for Ur III documents. According to Snell (ibid., 116), the silver implied with this formula is 1 gin2. This rendered the market rate, x unit quantity per 1 gin2 of silver (example: “9 sila3-ta” means “9 sila3 per (gin2 of silver).” This is seen here where the silver given (written ‘sum’) in problems 3, 11 – 13 and probably 9 is stated as 1 gin2 of silver per unit, as well as problems 5-8, 10, and probably 15-17, where 1 gin2 given per unit is implied. Only problem 14, a combined market rate exercise where the market rate is stipulated, differs from this formula (see §6.14). When identified as a rate per unit, we translate the suffix –ta as “per unit”; this unit is generally 1 gin2, thus, we can often translate as “per gin2.”
§2.2. sa10. ‘sa10’ is an interesting verb in these texts, and its exact understanding is essential for the interpretation of the problems. Neugebauer and Sachs first suggested the basic meaning of this verb as “to be equivalent,” with the nominalized form “equivalent” (written ‘šam2’ in MCT 97). This is taken up by Jacobsen who further illustrates its use in Sumerian texts and suggests a factitive meaning “to make equivalent.” ( Jacobsen 1946: 18). The use and development of sa10 is elaborated further in Steinkeller 1989: 153-162. When sa10 was used, a procedure was implied, namely, making the equivalence of one item, expressed as a quantity in a standard metrological system, to another item, expressed as a quantity in another standard metrological system. One literally made the equivalent. In the example of YBC 4607 (problems dealing with the volume of bricks) discussed by Neugebauer and Sachs in MCT p. 97, “i3 šam2 saḫar-bi,” is translated “oil, the (capacity-) equivalent of its (i.e. the brick’s) volume.” The reading sa10 rather than the conventional šam2 is consistent with Steinkeller’s interpretation (1989: 155). The translation would be then “the equivalent volume,” which makes sense in the context of the calculation of brick volumes, a context where oil is somehow incongruous (see Proust 2007: 214, and Friberg 2011: 262). In YBC 4698 problem 6 we see the operation of making equivalent played out with 30 gur of grain. The rate of equivalence is specified as (1 gin2 silver) per 1 gur grain.
The nuance, “to make equivalent” fits well with many of sa10’s appearances in YBC 4698. However, a sale of one good for a price in silver is, indeed, implied in the texts as well. Most striking in this regard is problem 6, just mentioned, which employs this verb together with the verb bur2 (see §2.3.) to mark a purchase and a sale respectively using silver as the medium of exchange. Moreover, problem 6 requests that one compute the gain in silver of the second transaction (represented by bur2). We see then, after the first use of sa10, the actor in the problem gains the right to sell the property acquired at a higher price and a payment is made in both transactions with a single medium of exchange: silver.
Merriam-Webster’s translates “buy” as: “1: to acquire possession, ownership, or rights to the use or services of by payment especially of money” and “4: to be the purchasing equivalent of.” Both uses of the term “to buy” are relevant here for sa10. As just seen, the acquisition of ownership, possession, and rights is certainly implied, and silver is used as the purchasing equivalent. This nuance of sa10 as “to buy” is supported by the Akkadian understanding of a purchase (Steinkeller 1989: 156-157) which the author of YBC 4698, as an Akkadian speaker, would most certainly have been aware of. We thus retain the use of “to buy” for sa10 in our translation, while making full note of the understanding “to make equivalent” for this verb.
§2.3. bur2. In problems #6-11 the verb bur2 is used opposite sa10 to denote the (re)sale of a specified product. The verb bur2, equated in the lexical tradition with the Akkadian verb pašāru has several different uses assigned to it often based on context (see, for instance, Sb Voc. II 170 presented in MSL 3, 142: bu-ur : bur2 = pa-ša2-ru ). In mathematical texts, the Akkadian verb pašārum can take on two different meanings: “to solve” (see Friberg 2007, 296: MS 3049 #1, line 9 where pa-šar is tentatively translated “solve”) or “to sell.”[9] The meaning to sell is played out in TMS 13: 4 where we read kî maṣi ašām u3 kî maṣi ap-šu-ur2, “at what price did I buy and at what price did I sell?” (see §6.9. below) pašāru is used to mark the (re)sale of the purchase marked by šâmum, the Akkadian equivalent of sa10 (see §2.2. above). We see a similar use of pašārum in TMS 13: 12 and perhaps 15; MLC 1842: 7; VAT 6469 i: 5; VAT 6546 ii: 7; and MS 3895 (Friberg 2010: 149). What we do not see, however, is the use of the Sumerian verb bur2 in any of these examples. Moreover, we are not aware of any instance where bur2 is employed in an economic or administrative text from the Old Babylonian period. Indeed, it seems to be an academic and literary term.
§2.4. ganba. This term corresponds to the Akkadian maḫīrum for which the CAD translates as “3. tariff, price equivalent, rate” (M1, 92, 94-97, especially 96-97 3f ). In mathematical texts, the ‘ganba’ of a given good is either the quantity of a good equivalent to 1 gin2 of silver (rate in-kind), or the equivalent in silver of 1 unit of a good (rate in-silver). The ganba is a key concept in economic mathematical texts.[10] Its translation as “market price” in mathematical texts is quite confusing for our purposes as it is actually an equivalency rate. We prefer to translate ‘ganba’ as “rate (in-kind)” or “rate (in-silver)” according to the context. Note that in #14-15, the value is estimated in-grain instead of in-silver.
We see this use born out in economic texts from the Old Babylonian period. For instance, a rate in-kind appears in HE 111 (RA 15, 191: 16-18) (among others) dated to the fifth year of Samsu-iluna of Babylon:
| 2(aš) sumsar šum-sikil-lumsar za-ḫa-din | 2 gur garlic, š.-plant, z.-plant, |
| ganba 3(barig)-ta | rate (in-kind) 3 barig per (gin2 silver), |
| ku3-bi 3 1/3 ˹gin2˺ | its silver value: 3 1/3 gin2 |
Moreover, a rate in-silver is clear in YOS 14, 290: 1-3 (NBC 8014) dated to Samsu-iluna year 6, where we read:
| ˹1/2˺ ma-na 6 1/2 gin2 ku3-babbar | 1/2 mana 6 1/2 gin2 silver, |
| sa10 3 2/3 gin2 ku3-sig17 | equivalent of 3 2/3 gin2 gold, |
| ganba-a 10 gin2-ta-am3 | rate in silver 10 gin2 per (gin2 gold) |
In both examples we note ganba’s appearance with the -ta (-am3) suffix to denote an equivalency rate.
§2.5. ib2-sa2. This verb corresponds to the Akkadian maḫārum, to make equal (see VAT 7530, obv. 10 and rev. 6). The procedure of making the quantities of various goods of different value (for example fine oil and common oil, iron and gold) equal is the basis of problems 3-5 and 12-14 of YBC 4698, as well as most other mathematical economic problems.
§2.6. e3 and e11. The expression “še ḫe2-e3 u3 ḫe2-e11-ma ganba ib2-sa2,” “let the grain rise and fall so that the rates are equal,” found in #14, is the exact parallel of the Akkadian expression “ku3-babbar li-li li-ri-id-ma ganba li-im-ta-ah-ra” found in VAT 7530, 19-20. A variant of the same expression appears as well in #15 (§6 below). This reading, which improves the understanding of the three last problems of the tablet, was suggested to us by Antoine Cavigneaux (personal communication).
§2.7. a-na. The term ‘a-na’ may be understood either as the Akkadian a-na (“to” or “for”), or Sumerian ‘a-na’ (corresponding to the Akkadian word mala and translated “as much as” or “as”). In the context of series texts, the second meaning is probable. Moreover, in our text, ‘a-na’ seems to connect two equivalent elements. For these reasons, we translate ‘a-na’ from Sumerian, following Thureau-Dangin,[11] although Akkadian ana cannot be excluded. However, note the use of ma-la in #16.
§2.8. Verbal forms. Here, as is often the case in mathematical series texts, verbal roots do not bear any grammatical element. This could lead one to leave the verbs unconjugated in the translation (as did Neugebauer in 1935; see MKT ch. 7 and Friberg 2005: 60). However, the alternation of conjugated and unconjugated Sumerian forms in series texts suggests that unconjugated roots may be abbreviations (see for example the case of AO 9072, analyzed in Proust 2009b: 171-172). Moreover, in the Old-Babylonian mathematical texts, generally written in Akkadian, the statements are almost always expressed in the first person singular simple past. Respecting the uses in Old-Babylonian mathematical tradition, we decided to translate the verbal forms in first person preterit, as did Thureau-Dangin (see for example TMB 148ff.), as well as Neugebauer from 1945 (see for example MCT 112ff.).
§2.9. Translation of units of measurement. In mathematical texts, the notations of units of measurement are highly standardized, and almost always appear as Sumerian ideograms (a few exceptions are found in texts from Diyala Valley). In modern publications, some of these units are translated (e. g. še is translated in English by grain, gin2 by shekel, ma-na by mina), while others are not (e. g. sila3, gur). This raises three problems. The first is consistency: if some of the units’ name cannot be translated, it is better to translate none of them. The second is the representation of ancient standardization: when units are translated in different modern languages (e. g. shekel in English, sicle in French), we artificially replace a unified ancient system by our Babel of language. The third and more important is the meaning of the modern translations: shekel is a unit of weight; however, in ancient texts, gin2 may represent not only a unit of weight (the sixties of one mana), but also a unit of capacities (the sixties of one sila3), or surface (the sixties of one sar). Thus, following Neugebauer and Sachs in MCT, we decided to leave the Sumerian names of the units untranslated.
§3. Methodological remarks
§3.1. Our text contains only statements of problems, without procedure for resolution, and, often, with neither question nor answer. Thus, the goal of the problems is not always explicit. However, the full understanding of the statement requires the reader to solve the supposed problem. As much as possible, we tried to implement calculations leading to the solution. This raises a methodological issue: what is the historical relevance of calculations for which we have little trace in our text? This question refers to a symmetrical one: in the corpus of mathematical cuneiform texts, we find a lot of school exercises with numerical calculations, but without indication of their goal. These exercises probably correspond to statements of problems which are absent from the text. Thus, we have on the one hand, statements without resolution in series texts, and on the other hand, resolutions without statements in some school texts. Of course it would be highly speculative to decide that some of the school exercises correspond to the resolution of some of the statements found in series texts. Even so in some cases, given the similarities of numerical data, we may be tempted to do so. The important point for us is that some school exercises present a specific pattern offering a powerful tool for solving mathematical economic problems. This pattern uses a tabular format to represent the algorithm for making equivalences of values, with fixed sequences of operations represented by columns.
| Col. | I | II | III | IV |
| Reciprocal of I | II × 28.48 | I × III | ||
| Rate in-kind | Rate in-silver | Value in-silver | Value in-kind | |
| Good 1 | 1 | 1 | 28.48 | 28.48 |
| Good 2 | 2 | 30 | 14.24 | 28.48 |
| Good 3 | 3 | 20 | 9.36 | 28.48 |
| Good 4 | 4 | 15 | 7.12 | 28.48 |
| Total | 2.5 | 1 | ||
| Table 1: tabular presentation of exchange problems based on data of MS 2830 | ||||
§3.2. Interesting examples of such “combined market rate exercises” were analyzed by Friberg (2007: 157-168). Eight of such exercises noted on “square tablets,” kept at Yale University, were published in MCT 17, and gathered again by K. Nemet-Nejet (2002: 253-258) with photos.[12] These “combined market rate exercises” were not necessarily produced in the same mathematical context as series texts. However, they offer a relevant framework of calculation for solving most of the problems of YBC 4698. Thus, instead of implementing calculations and reasonings based on modern algebraic or arithmetic methods, we try to solve problems of our tablet by using the tabular presentation, attested in related school texts. The striking parallel between YBC 4698 and “combined market rate exercises” was first underlined by Friberg (2005: 60-61). However, Friberg does not utilize this parallel to implement his own calculation. This entails a different approach for interpretation. For example, the interpretation of problems 3 and 6-10 by Friberg is mathematically equivalent to ours, but the manner in which the calculations are explained is not the same.
§3.3. The examples provided by Friberg are purely numerical texts, without any word indicating the context, except MS 2830, which states the total value in silver by mention of “1 gin2 ku3-babbar” (1 gin2 of silver) on the bottom edge.[13] The first section of the reverse of MS 2830 reads as follows:
| 1 gin2 ku3-babbar | |||
| 1 | 1 | 28.48 | 28.48 |
| 2 | 30 | 14.24 | 28.48 |
| 3 | 20 | 9.36 | 28.48 |
| 4 | 15 | 7.12 | 28.48 |
The relationships between data of the four columns are the following:
Column II contains the reciprocals of column I.
Column III contains the product of the values of column II by a constant coefficient (here 28.48).
Column IV contains the product of values of column I by the values of column III (that is, the coefficients of column II; thus, these products are equal).
The total of values of column III is 1, that is the number in sexagesimal place value notation corresponding, in metrological tables (see outline in §8.3), to the value “1 gin2 of silver” noted on the bottom edge.
Diverse mathematical situations may be represented by these relationships. The observation of interest for us is that these situations are described in many sections of the tablet YBC 4698.
§3.4. According to various parallels presented by Friberg, including YBC 4698, the meaning of the rows and the columns of the first section of the reverse of MS 2830 may be the following:
Each row corresponds to a given good (named as good 1, good 2, etc. in Table 1).
Column I contains the rate in-kind of the goods, that is, the quantity equivalent to 1 gin2 of silver.
Column II contains the reciprocal of rates in-kind, that is, the rate in-silver of the goods, or, in other terms, the value in silver of 1 unit (generally, 1 sila3) of the goods.
Column III contains the value in silver of the actual quantity of the goods (of which the total is usually 1 gin2).
Column IV contains the actual quantities of the several goods which have been made equal.
Moreover, an important feature of the table is that the values provided in each cell are expressed in sexagesimal place value notations (henceforth SPVN).
§3.5. It is important for the following calculations to note that the relationships between columns are not always transmissible to sums and differences: they are for multiplication by a coefficient (for example, items of column III are the products of items of column II by a coefficient, and it is the same for the sum), and are not for reciprocals and products of columns (for example, items of column II are the reciprocals of items of column I, but the sum of column II is not the reciprocal of the sum of column I).
§3.6. Many of the problems of our tablet are structured according to a unique mathematical framework, represented by Table 1 or variants. A similar framework can be used as well for completely different mathematical situations, for example sets of rectangles of equal area. In this case, column I contains the widths of the rectangles, column II the reciprocals of the widths, column III the lengths, and column IV the equal areas (see examples in Proust 2007: 202-205).
§3.7. Organization of our calculations. In our commentary of YBC 4698, in particular in the reconstruction of the calculations expected for solving the problems (§6), we attempt, as much as possible, to follow the mathematical framework attested in OB mathematical texts, especially in school texts. This implies the following chart to represent the entire mathematical framework:
- The metrological data provided by the statements of the problems are transformed into SPVN according to the metrological tables. Thus, the reader is invited to refer to these metrological tables throughout the reading of this article (see §8.3).
- The calculations in SPVN are represented in tabular form, where each column represents an operation (for example, column II represents reciprocals of data of column I, or column III represents the multiplication by a coefficient of data of column II, and so on).
- The results of the calculations are transformed into measures according to the metrological tables, with a mental control of the orders of magnitude.
It is important to keep in mind that this framework includes both the use of metrological tables and tabular presentation of numbers in SPVN. When relevant from a mathematical point of view, we use the three-step chart above in our reconstructions of the possible calculations performed by ancient scribes (see conventions below in §3.8). We prefer to use charts attested in Old Babylonian sources instead of modern formalism because we are convinced that the organization of calculations made by modern historians has a substantial impact on the understanding of ancient texts. This impact is as important (and even more so) as the choice of notations in the transliterations, or the terminology used to translate the ancient terms.
§3.8. Conventions. To be clear in the tabular presentation, to distinguish the data provided explicitly or implicitly by the original text from the results of our calculations, we note in bold the former and in plain the latter. Correspondences between metrological data and abstract numbers as provided by metrological tables are represented by an arrow (→). For example: 1 gin2 → 1, or 1 še → 20. An outline of the metrological tables can be found in §8.3, and the complete set of metrological tables attested in Nippur is provided in Proust 2009.
§4. Transliteration and translation
| obverse i | |||
| 1 | 1 | še a-na 1(aš) še gur | (When the principal in) grain (is) as much as 1 gur of grain, |
| 2 | 1(barig) še a-na maš2 šum2 | 1 barig of grain as the interest I give. | |
| 3 | še u3 maš2 en-nam | The grain and the interest are how much? | |
|
|
|||
| 2 | 4 | še a-na 1(aš) še gur | (When the principal in) grain (is) as much as 1 gur of grain, |
| 5 | 1(barig) 4(ban2) a-na maš2 šum2 | 1 barig 4 ban2 as the interest I give. | |
| 6 | še u3 maš2 en-nam | The grain and the interest are how much? | |
|
|
|||
| 3 | 7 | 3 sila3-ta i3 sag | (Rates in-kind are) 3 sila3 of first quality oil per (gin2) |
| 8 | 1(ban2) 2 sila3-ta i3-geš | (and) 1 ban2 2 sila3 of common oil per (gin2). | |
| 9 | 1 gin2 ku3-babbar šum2 | 1 gin2 of silver I gave. | |
| 10 | i3-geš u3 i3 sag | Common oil and first quality oil | |
| 11 | ib2-sa2-ma sa10 | I made equal and I bought. | |
|
|
|||
| 4 | 12 | 1(geš2) 3(u) DUG an-bar | 60+30 DUG of iron |
| 13 | 9? DUG ku3-sig17 | 9 (×60?) DUG of gold, | |
| 14 | 1 ma-na ku3-babbar šum2 | 1 mana silver I gave. | |
| 15 | an-bar u3 ku3-sig17 | The iron and gold | |
| 16 | 1 gin2-ma sa10 | (is) 1 gin2 and I bought. | |
|
|
|||
| 5 | 17 | 1/2 ma-na ku3-babbar šum2 | 1/2 mana of silver I gave. |
| 18 | 1(aš) gur-ta sa10 | 1 gur per (gin2) I bought. | |
|
|
|||
| obverse ii | |||
| 6 | 1 | 3(u) še gur | 30 gur grain |
| 2 | 1(aš) še gur-ta sa10-ma | 1 gur of grain per (gin2) I bought, | |
| 3 | 4(barig) še-ta bur2-ra | 4 barig of grain per (gin2) I sold. | |
| 4 | ku3 diri en-nam | The silver profit is how much? | |
| 5 | 7 1/2 gin2 ku3-babbar diri | 7 1/2 gin2 of silver is the profit. | |
|
|
|||
| 7 | 6 | sag ku3-bi en-nam | The initial price is how much? |
|
|
|||
| 8 | 7 | 1(aš) gur i3-geš | 1 gur of common oil. |
| 8 | 1(ban2)-ta sa10-ma | 1 ban2 per (gin2) I bought and, | |
| 9 | 8 sila3-ta bur2-ra | 8 sila3 per (gin2) I sold. | |
| 10 | ku3 diri en-nam | The silver profit is how much? | |
| 11 | 7 1/2 gin2 ku3 diri | 7 1/2 gin2 is the silver profit. | |
|
|
|||
| 9 | 12 | 1(aš) gur i3-geš | 1 gur of common oil. |
| 13 | i-na sa10 1! gin2 | When the purchase is 1! (text: 2) gin2, | |
| 14 | 2 sila3 šuš4 | 2 sila3 I cut. | |
| 15 | 7 1/2 gin2 ku3 diri | 7 1/2 gin2 is the profit. | |
| 16 | en-nam sa10-ma | How much did I buy? | |
| 17 | en-nam bur2-ra | How much did I sell? | |
| 18 | 1(ban2) sa10-ma | 1 ban2 I bought and | |
| 19 | 8 sila3 bur2-ra | 8 sila3 I sold. | |
|
|
|||
| reverse i | |||
| 10 | 1 | 1(aš) gur i3-geš | 1 gur of common oil. |
| 2 | 9 sila3-ta sa10-ma | 9 sila3 per (gin2) I bought, | |
| 3 | 7 1/2 sila3 bur2-ra | 7 1/2 sila3 per (gin2) I sold. | |
| 4 | ku3 diri en-nam | The profit is how much? | |
| 5 | 6 2/3 gin2 ku3 diri | 6 2/3 gin2 is the silver profit. | |
|
|
|||
| 11 | 6 | 1(aš) gur i3-geš | 1 gur of common oil. |
| 7 | i-na sa10 1 gin2 1 1/2 sila3 šuš4 | When the purchase is 1 gin2, 1 1/2 sila3 I cut. | |
| 8 | 6 2/3 gin2 ku3 diri | 6 2/3 gin2 is the silver profit. | |
| 9 | en-nam sa10-ma en-nam bur2-ra | How much did I buy? How much did I sell? | |
| 10 | 9 sila3 sa10-ma 7 1/2 sila3 bur2-ra | 9 sila3 I bought, and 7 1/2 sila3 is sold. | |
|
|
|||
| 12 | 11 | 7 ma-na u3 11 ma-na siki geš? | (Per) 7 mana and 11 mana of ... wool |
| 12 | 1 gin2 ku3 šum2 ib2-sa2-ma sa10-ma | 1 gin2 silver I paid. I made equal and I bought. | |
| 13 | 4.16.40 sa10 | 4.16.40 I bought. | |
|
|
|||
| 13 | 14 | 7 sila3-ta i3-geš | Per 7 sila3 of common oil |
| 15 | 1(ban2) 2(diš) sila3-ta i3-šaḫ2 | per 1 ban2 2 sila3 of lard, | |
| 16 | 1(diš) gin2 šum2 ib2-sa2-ma sa10 2(ban2) 3 1/3 sila3 | 1 gin2 I gave. I made equal and I bought: 2 ban2 3 1/3 sila3. | |
|
|
|||
| 14 | 17 | ganba a-na 1 2 3 4 5 | (When) rates (in-kind are) as much as 1 2 3 4 5 |
| 18 | 6 7 8 9 ganba | 6 7 8 9, the rate (in grain) | |
| 19 | 1(barig) še šum2 še he2-e3 | 1 barig I give. Let the grain rise | |
| 20 | u3 he2-e11-ma | or fall so that | |
| 21 | ganba ib2-sa2 | the rates (in-kind) are equal. | |
|
|
|||
| reverse ii | |||
| 15 | 1 | ganba 3 ku6-a 5 sila3 | Rate (in-kind) for 3 fish, 5 sila3 |
| 2 | 5 ku6-a ku6 [...] | for 5 fish, fish ... | |
| 3 | še gar-gar-ma 4(aš) 1(barig) 4(ban2) 5 (2?) < sila3 gur> |
The grain I added: 4 (gur) 1 barig 4 ban2 5 (2?) (sila3 gur) | |
| 4 | ku6-a gar-gar-ma ku6 | The fish I added. Let the fish | |
| 5 | še ḫe2-e3 u3 ḫe2-e11-ma | and the grain rise or fall | |
| 6 | ku6-a ib2-sa2 | so that the fish are equal. | |
|
|
|||
| 16 | 7 | ma-la ganba ḫi-a nagga | As much as the rates (in-kind) of the lead |
| 8 | ku3 i3?-la2-ma | silver I weighed and | |
| 9 | nagga sa10 | lead I bought. | |
| 10 | ku3 u3 nagga gar-gar-ma | The silver and the lead I added: | |
| 11 | 7 ma-na ku3 u3 nagga 7 mana. | The silver and the lead | |
| 12 | en-nam | are how much? | |
|
|
|||
| 17 | 13 | na4 šu-ti-a-ma | Stones I received and |
| 14 | ki-la2-bi nu-zu | their weight I don’t know. | |
| 15 | 6-ta sa10 | 6 (stones) per (gin2) I bought. | |
| 16 | 6-ta ku3-sig17 gar-ra | 6 gold inlaid (stones) per (gin2 I bought). | |
| 17 | ku3-sig17 gar-ra | Gold inlaid (stones), | |
| 18 | a-na 6-ta sa10 | as much as 6 (stones) per (gin2) I bought. | |
| 19 | na4 ku3-babbar en-nam | The stone, the silver how much | |
| 20 | gar-gar-ma 1/2 ma-na 1 1/2 gin2 | did I add? 1/2 mana 1 1/2 gin2 | |
| 21 | x ma na? gar-gar-ma | ... I added and | |
| 22 | 1 ma-na 3 gin2 | 1 mana 3 gin2. | |
|
|
|||
| bottom edge | |||
| 1 | (erasure) dub 3-kam-ma | Tablet number 3. | |
§5. Philological notes
| #3 | In obv. i 3 and passim, we read i3, following Thureau-Dangin (1937b: 89) and Friberg (2005: 61), but Neugebauer reads NA4 under Goetze’s suggestion (see Archives Aaboe-Britton in §9 below). In obv. i 11 and passim, we read sa10 following Thureau-Dangin (1937b: 89) and Friberg (2005: 61) who chooses the nominalized form šam2, but Neugebauer reads ANŠE. |
| #4 | In obv. i 12-13, we read “DUG,” following Neugebauer (MKT 3, 42), which is confirmed by our collations as well as a reading performed by Cavigneaux. However, Friberg (2005: 61) reads “-bi,” thus, his interpretation is completely different (ibid., 67-68 and §6.4 below). We may understand this situation as one dealing with objects, the nature of which we cannot currently identify, made of iron and gold. Since iron was a very rare and precious metal in the Old Babylonian period, it is surprising that the proportion of iron and gold is 90 to 9. However, it is possible that the proportion is 90 to 9×60 (or 1 to 6). Thus 9 may represent 9 sixties instead of 9 units (according to another suggestion of Cavigneaux). In this case, we should restore “9 <šu-ši>.” Another possibility would be that “DUG” refers to a container, perhaps alluding to leftovers from the metallurgical process. For a textual example of this latter use of a metal, see YOS 2, 112 11, an-na ḫi-im-mi u3 ša-ak-ti-šu, which we translate “tin sweepings and its powder.” |
| #5 | In obv. i 18, we read “1(aš) gur-ta,” following Neugebauer (MKT 3, 42), but Friberg (2005: 61) reads “1/2 gin2-ma.” |
| #6 | In obv. ii 3 and passim, we read “bur2” following Friberg (2005: 61) and Cavigneaux (pers. com.), but Neugebauer (MKT 3, 42) reads “bala.” |
| #9 | In obv. ii 14 and passim, we read the sign “ŠUM” following Neugebauer (MKT 3, 42), which we transliterate as “šuš4,” to cut, because of the parallel with kašāṭum, to cut, found in an analogous text, TMS 13. However Friberg (2005: 61) reads “si3.” |
| #12 | In rev. i 11, we read “siki geš?” (wool of a certain kind) where Neugebauer reads (MKT 3, 42) “RU …,” and Friberg (2005: 61) “ru-qa.” |
| #13 | In rev. i 14, Friberg and Neugebauer read 6 sila3. However, we distinguished clearly the number “7” on the tablet. In line 15, the last sign is probably sag, as in other similar contexts, but this sign is not clear; Friberg reads “šaḫ.” In rev. i 16, the signs 2 ban2 3 1/3 sila3 are visible on the right edge of the tablet, but they do not appear in Neugebauer’s copy, nor in Friberg’s transliteration (Friberg 2005: 61). According to the Archive Aaboe-Britton now kept at ISAW (see §9), Neugebauer used the photos of the obverse and reverse of the tablet, but did not have the photos of the edges, while Friberg’s analysis of YBC 4698 was based solely on Neugebauer’s hand copy. |
| #14 | The reading of this statement by Friberg is quite far from ours (and from Neugebauer’s): in rev. i 18, Friberg reads “šu-ši” instead of “ganba”; line 19 he reads “1 še si3 še šam2-ma” instead of Neugebauer’s “1 gun2 sum še ib2-sa2 du6.” Following Neugebauer, however, he reads “GIŠ” instead of “DU” in line 20. In the beginning of rev. i 19, the reading 1(barig) še is probable, but not certain. Neugebauer (MKT 3, 42) transliterates “3(gur)(?),” which seems quite improbable, and Friberg (2005: 61) “1 (gur) še,” which is possible, but not consistent with the other data of the statement. |
| #15 | In “ḫe2-e3 u3 ḫe2-e11” (rev. ii 5), the reading of e11 (DU6.DU) is uncertain. There is the possibility of reading this e3 (UD.DU), though taken with problem 14 ll. 19-20 it is clear that e11 was intended by the scribe. Note that the -ma placed in line 6 belongs in fact to line 5 that lacked space for it. In rev. ii 4, we believe that the last sign is clearly ‘ku6,’ while Friberg (pers. comm.) suggests another possibility: “this should be a number, possibly 2 ner = 20.00.” We exclude this possibility because the graphy of the sign here is the same as in ll. 1 and 2 of this section, and the number “2(geš’u)” should have a slightly different graphic form (wedges and Winkelhaken more neatly separated—see for example HS 1703 rev. v). Moreover, we do not expect a number noted in system S here. |
| #17 | Rev. ii 16-17, we translate ‘ku3-sig17 gar-ra’ as ‘gold inlaid,’ following Cavigneaux’s suggestion. |
§6. Comments
| #1 | The statement gives the interest to be paid for the loan of a given amount of grain that is, 1 barig per gur. The question asks principal and interest, or, perhaps, the total principal + interest. It seems that some information concerning the principal is missing, so we cannot solve the problem. Perhaps the missing data were given in previous tablets of the series. Or, as another hypothesis, suggested by Neugebauer (MKT 3 43), the question concerns the interest rate, that is, the interest by unit of grain (1 sila3). In this case, the calculation would be quite simple. |
| 1 gur | → 5 | |
| 1(barig) | → 1 |
| The interest rate is thus 1/5, that is, 12, which corresponds to 12 gin2 per sila3. Note that the interest rate of 1/5 (or 20%) is the standard value for annual silver loans. The customary value for grain is 1/3 per year though texts do vary. See, for instance, YOS 14, 178: 2, a loan where the interest rate is stipulated at 1 barig per gur, the rate presented here. Interestingly, Friberg (personal communication) suggests an even simpler solution, that is, 1 gur is the actual principal, 1 barig is the actual interest, and the question is “what is the principal plus (u3) the interest.” The answer should be 1 gur 1 barig. |
| #2 | This statement is identical to the previous one, with an interest of 1 barig 4 ban2 per gur. The calculation of the interest rate would be: |
| 1 gur | → 5 | |
| 1(barig) 4(ban2) | → 1.40 |
| The interest rate is thus 1.40/5, that is, 1.40 × 12 = 20, which corresponds to 20 gin2 per sila3. Note that this corresponds to a rate of 1/3, which is the customary rate for a grain loan as stated above. |
| #3 | The rate in-kind of first quality oil is given as 3 sila3 (this means that the value of 3 sila3 of first quality oil is 1 gin2 of silver), and the rate in-kind of common oil is given as 1 ban2 2 sila3 (the value of 1 ban2 2 sila3 of common oil is 1 gin2 of silver). The same quantity of both oils is bought. However, this common quantity is not specified, and there is no question, nor answer, so that we may consider the following hypothesis: The total value in silver of the oils bought is 1 gin2 (line 9). The problem can be solved by using the chart as explained in §3: the metrological data are converted into SPVN using metrological tables, the numbers in SPVN are displayed in tabular format and finally, the result is converted into metrological notation by means of a metrological table. Note that in Table 2 and thereafter, bold numbers correspond to data provided by the text, either as SPVN, or as metrological notation, while the use of metrological tables is represented by an arrow (→). Here, metrological tables provide: |
| 3 sila3 | → 3 | |
| 1(ban2) 2 sila3 | → 12 | |
| 1 gin2 | → 1 |
| The reader has to calculate the value of each kind of oil (column III of Table 2), and/or the common quantity (column IV). To solve the problem, we first note that the rate in-silver (column II) is the reciprocal of the rate in-kind (column I) and fill in accordingly. The total of items of column II is 25, and the total of the items of column III is 1. Thus, the coefficient of column III is 2.24 (reciprocal of 25) and column III is easy to fill. We can then fill in column IV which is the product of data of columns I and III, that is, the coefficient 2.24. According to metrological tables and a mental control of the orders of magnitude, the results correspond to the following weights and capacity: |
| 48 | → 2/3 gin2 24 še | |
| 12 | → 1/6 gin2 6 še | |
| 2.24 | → 2 1/3 sila3 4 gin2 |
| For 2 1/3 sila 4 gin2 of premium oil, 2/3 gin2 24 še silver is given and for the same amount of common oil, 1/6 gin2 6 še silver is given. This seems to be the solution espoused by Friberg (2005: 60-61). |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 2.24 | I × III | |||
| Rate in-kind | Rate in-silver | Value in-silver | Value in-kind | ||
| First quality oil | 3 | 20 | 48 | 2.24 | |
| Common oil | 12 | 5 | 12 | 2.24 | |
| Total | 25 | 1 | |||
| Table 2: calculations for solving #3 | |||||
| #4 | Statement #4 seems to be similar to #3, except for two key points: the suffix –ta is not used nor does it state that the two goods “I made equal and I bought” but instructs that the two goods (here iron and gold) “is 1 gin2 and I bought,” in the same position. Like in #3, the transaction is expressed by the verbs ‘sum’ (to give) and ‘sa10’ (to buy). However, this transaction is not completely clear to us. Only an attempt of interpretation is offered here. |
| Lines 12-13 seem to indicate that we deal with two lots of objects (DUG): 90 (60+30) iron objects and 9 gold objects. Line 14 states “1 mana silver I gave,” thus we understand that 1 mana is the total value in-silver of the two lots, like in #3. Lines 15-16 refer to ‘1 gin2’ in relation to iron and gold. The nature of this relation is not clear. 1 gin2 can hardly be the total weight of the two lots, or its total value in-silver (a weight of about 8 g for 99 objects is hard to imagine; and a value in-silver of 8 g for these 99 precious objects is even more unrealistic). We can guess that 1 gin2 represents the weight of each object. Even so, this is not made explicit in the text. If this suggestion is correct, the total weight of iron bought is 1 . mana (90 times 1 gin2) and the total weight of gold bought is 9 gin (9 times 1 gin2). The goal of the problem may be the value in silver of the 90 iron objects and of the 9 gold objects. Thus we need to know the rate in-silver of both metals. It then seems that data needed to evaluate rates is missing. This data could have been well known by the reader of the text, or it may have been provided in previous tablets of the series. Note that, if we suppose that the rate in-silver of iron is 8, a widely attested value (see below), then the rate in-silver of gold is 5.20 (because we know that the total value of iron plus gold is 1 mana). 5.20 is a realistic value for the rate in-silver of gold. Moreover, the rates 8 and 5.20 are regular numbers, as well as their sum (13.20), and the sum of their reciprocals 7.30 and 11.15 (18.45). The understanding of the problem by Friberg (2005, 67), is different. For him, “iron and gold are 90 and 9 times more valuable than silver, and if 1 shekel of iron and gold together is bought for 1 mina of silver, what are then the amount of iron and gold, respectively?” In modern language, this should lead to a system of two linear equations. The solution should be 37.46.40 and 22.13.20 (;37 46 40 and ;22 13 20 with Friberg’s notations). We do not believe that the first two lines of #4 state that “iron and gold are 90 and 9 times more valuable than silver,” because the syntax of the cuneiform text should be different. We should have: “1(geš2) 3(u) gin2-ta an-bar / 9(diš) gin2-ta ku3-sig17.” Moreover, the values would be completely unrealistic. According to Cécile Michel (pers. comm.), “In the Old Babylonian period, the ratio iron : silver is 1:8 in Southern Mesopotamia; 1:12 in Mari, and 1:40 in Aššur. The ration gold : silver is between 1:3 and 1:6 in Southern Mesopotamia, between 1:4 and 1:6 in Mari, and between 1:4 and 1:8 in Aššur.” |
|
| #5 | Problem #5 seems to be a variant of #4. According to a system of notation widely used in series texts, only the modified information is noted in the variant, the unchanged information being omitted. The complete statement of #5 would be the following (the information given in #4 but omitted in #5 is noted between triangular brackets): |
| <1(geš2) 30 DUG an-bar> | |
| <9(geš2?) DUG ku3-sig17> | |
| 1/2 ma-na ku3-babbar sum | |
| 1(aš) gur-ta sa10 |
| The variant should be: The total value in-silver of the two lots is 1/2 mana instead of 1 mana The weight in gin2 is replaced by an equivalent value in-grain (which means that the weight of iron objects is not the same as the weight of gold objects). The interpretation raises the same difficulties already witnessed in #4. |
| #6 | Neugebauer interpreted the statement as asking for a profit made in purchasing 30 gur of grain, knowing expected losses (5 barig), and actual losses (4 barig) (MKT 3, 44). The situation is much simpler if we consider that the transaction consists in buying and selling 30 gur of grain, and that the question asks for the profit in silver. This is also the understanding of Friberg (2005: 217), against Neugebauer. The text states that if 1 gur of grain is bought for a given price (sa10), 4 barig of this grain is sold (bur2) for the same price. Data consistency requires that the purchase price of 1 gur of grain is 1 gin2 of silver, but this value is not provided in the statement. Indeed, since the market prices are usually given as capacity by gin2 (rate in-kind) it is not necessary to precise “per 1 gin2,” which is implicit. This interpretation assumes that bur2 here has the sense ‘to sell,’ that is, the contrary of to buy (reverse operation, see §2.3). The calculations may run as follows. First, the metrological data are transformed into SPVN using the metrological table of capacities: |
| 30 gur | → 2.30 | |
| 1 gur | → 5 | |
| 4(barig) | → 4 |
| Then, the calculations are performed with the aid of tabular format indicated in table 3. Finally, the profit is 7.30, the difference between the sale and purchase prices (column III). The metrological table of weights provides the metrological expression of the profit: |
| 7.30 | → 7 1/2 gin2 |
| The profit is 7 1/2 gin2, as stated in the answer (obv. col. ii, line 5). Note that the profit (7.30) may be calculated in two ways: |
| a) Multiplying the difference of col. II (3) by the coefficient 2.30 | |
| b) Calculating the difference of purchase and selling prices in col. III. |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 2.30 | I × III | |||
| Rate in-kind | Rate in-silver | Price in-silver | Value in-kind | ||
| Purchase | 5 | 12 | 30 | 2.30 | |
| Sale | 4 | 15 | 37.30 | 2.30 | |
| difference | 3 | 7.30 | |||
| Table 3: calculations for solving #6 | |||||
| #7 | This section seems to be an additional question related to the statement of #6. If we consider that the situation is the same as in #6, the additional question probably asks the purchase price of the 30 gur of grain. This is an indication that, in #6, the profit is calculated by method a). The purchase price of the 30 gur is obtained by multiplying 12 by 2.30 (see Table 3). The result is 30, which corresponds to a purchase price of 30 gin2. |
| #8 | This problem is similar to #6, but the good purchased is 1 gur of oil instead of 30 gur of grain. As in the previous section, the metrological data are transformed into SPVN using the metrological table of capacities: |
| 1 gur | → 5 | |
| 1(ban2) | → 10 | |
| 8 sila3 | → 8 |
| Then, the calculations are performed with the aid of tabular format indicated in table 4. Finally, the profit is 7.30, the difference of the sale and purchase prices (column III). The metrological table of weights provides the metrological expression of the profit: |
| 7.30 | → 7 1/2 gin2 |
| The profit is 7 1/2 gin2, as stated in the answer (obv. ii 11). |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 5 | I × III | |||
| Rate in-kind | Rate in-silver | Price in-silver | Value in-kind | ||
| Purchase | 10 | 6 | 30 | 5 | |
| Sale | 8 | 7.30 | 37.30 | 5 | |
| difference | 1.30 | 7.30 | |||
| Table 4: calculations for solving #8 | |||||
| #9 | A new operation appears in line 14: 2 sila3 are “cut” (šuš4) from the good sold. This translation of ‘šuš4’ is suggested by TMS 13, which contains a similar problem with a complete resolution. The statement TMS 13 (lines 1-4) runs as follows, according to our collation of the tablet, which corresponds to Friberg’s transliteration (2010: 154), except in line 2, where Friberg left out the sequence ‘i3-geš’. |
| 2(gur) 2(barig) 5(ban2) i3-geš sa10 i-na sa10 1 gin2 ku3-babbar | 2 gur 2 barig 5 ban2 of common oil I bought. When the purchase (costs) 1 gin2 silver (of oil), | |
| 4 sila3-ta-am3 i3-geš ak-ši2-iṭ-ma | 4 sila3 of common oil I cut and | |
| 2/3 ma-na 20 še ku3-babbar ne-me-la | 2/3 mana 20 še silver of profit I see. | |
| a-mu-ur2 ki ma-ṣi a-ša-am u3 ki ma-ṣi ap-šu-ur2 | How much did I buy? How much did I sell? |
| Parallel terms in TMS 13 and YBC 4698, resp.: |
| kašāṭum | šuš4 | to cut | |
| šâmum, sa10 | sa10 | to buy | |
| pašārum | bur2 | to sell |
| ‘šuš4’ is parallel to the Akkadian ‘kašāṭum’ used in TMS 13. The 2 sila3 “cut” represents the difference between the purchase and the selling rates in-kind. |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 5 | I × III | |||
| Rate in-kind | Rate in-silver | Price in-silver | Value in-kind | ||
| Purchase | 10 (a) | 6 (a') | 5 | ||
| Sale | 8 (b) | 7.30 (b') | 5 | ||
| difference | 2 | 1.30 | 7.30 | ||
| Table 5: calculations for solving #9 | |||||
| Thus, we can understand the statement of YBC 4698 #9 as a reverse problem of the previous one: the difference between the purchase and the selling rates in-kind being 2 sila3, and the profit for 1 gur being 7 1/2 gin2, find the purchase and the selling rates in-kind. However, this interpretation assumes that the purchase rate is given per 1 gin2, not per 2 gin2 as noted in the tablet (obv. col. i, line 13) |
| 1 gur | → 5 | |
| 2 sila3 | → 2 | |
| 7 1/2 gin2 | → 7.30 |
| Since the difference of price in-silver is 7.30 (col. III of table 5), the difference of rates in silver is 7.30/5, that is, 1.30 (col. II). The problem consists, then, of finding two numbers, a and b, from their difference (2) and the difference of their reciprocals a' and b' (1.30). In modern language, this problem may be represented by the system of equations: |
| a – b | = 2 | |
| b' – a' | = 1.30 |
| This leads to the problem of finding two numbers knowing their difference (2) and their product (2/1.30, that is, 1.20) (for the method of resolution of this quadratic problem, see the explanation of TMS 13 in Høyrup 2002: 206-209). The solution is a = 10 and b = 8. |
| 10 | → 1(ban2) | |
| 8 | → 8 sila3 |
| The purchase and the selling rates in-kind are thus 1 ban2 and 8 sila3. This solution corresponds to the answer given in the text (obv. col. ii, lines 18-19). | |
| #10 | This problem is similar to #8, with other purchase and sale rates. The purchase and the selling rates in-kind are 9 sila3 and 7 1/2 sila3. The metrological data are (in SPVN): |
| 1 gur | → 5 | |
| 9 sila3 | → 9 | |
| 7 1/2 sila3 | → 7.30 |
| See table 6 for calculations. The result 6.40 provided by col. III, corresponds to 6 2/3 gin: |
| 6.40 | → 6 2/3 gin2 |
| The profit is 6 2/3 gin2, as stated in the answer (rev. i 5). |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 5 | I × III | |||
| Rate in-kind | Rate in-silver | Price in-silver | Value in-kind | ||
| Purchase | 9 | 6.40 | 33.20 | 5 | |
| Sale | 7.30 | 8 | 40 | 5 | |
| difference | 1.20 | 6.40 | |||
| Table 6: calculations for solving #10 | |||||
| #11 | As in the case of #9, the problem #11 presents the reverse of the previous one. |
| 1 gur | → 5 | |
| 1 1/2 sila3 | → 1.30 | |
| 6 2/3 sila3 | → 6.40 |
| Since the difference of prices in-silver is 6.40 (col. III of table 7), the difference of rates in-silver is 6.40/5, that is, 1.20 (col. II). The problem consists then, of finding two numbers, a and b, from their difference (1.30) and the differences of their reciprocals a' and b' (1.20). In modern language, this problem may be represented by the system of equations: |
| a – b | = 1.30 | |
| b' – a' | = 1.20 |
| The solution of this quadratic problem is a = 9 and b = 7.30. |
| 9 | → 9 sila3 | |
| 7.30 | → 7 1/2 sila3 |
| The purchase and the selling rates in-kind are thus 9 sila3 and 7 1/2 sila3. This solution corresponds to the answer given in the text (rev. i 10). |
| Col. | I | II | III | IV | |
| Reciprocal of I | II × 5 | I × III | |||
| Rate in-kind | Rate in-silver | Price in-silver | Value in-kind | ||
| Purchase | 9 (a) | 6.40 (a') | 5 | ||
| Sale | 7.30 (b) | 8 (b') | 5 | ||
| difference | 1.30 | 1.20 | 6.40 | ||
| Table 7: calculations for solving #11 | |||||
| #12 | Problem 12 provides the rate in-kind of two types of wool, 7 mana and 11 mana respectively, as well as their total value in silver, 1 gin2. There is no question, but it seems that the problem is to find the equal quantities of wool 1 and wool 2, and that the answer is given in the last line: this quantity corresponds to 4.16.40. In this problem, the rates in-kind correspond to non-regular numbers (7 and 11), which means that the reciprocal cannot be found. One finds the same situation in VAT 7530, as well as in several “combined market rate exercises” (see Friberg 2007: 162, 165). These texts suggest that, as the reciprocal of the rates in-silver cannot be found, column II does not provide the rates in-silver, but instead the product of the rates in-silver by 7 ×11. |
| 7 ma-na | → 7 | |
| 11 ma-na | → 11 | |
| 1 gin2 | → 1 |
| Column II of table 8 contains the product of the reciprocal of 7 by 7×11, which is 11, and the product of the reciprocal of 11 by 7×11, which is 7. The total of column II is 18. The number 18 is a false value which must be compared to the actual total value in silver of 1 gin2 (total column III) in order to produce a coefficient of 3.20 (the reciprocal of 18). This coefficient is necessary to multiply the rates in column II in order to produce column III. Thus we see: |
| 11× 3.20 | = 36.40 | |
| 7 × 3.20 | = 23.20 |
| The next step is to multiply the rates in column I by the values in silver in column III to produce the values in kind of column IV, 4.16.40 in both cases. Thus we have filled in the table and obtained the required equal quantities of wool 1 and wool 2: |
| 4.16.40 | → 4 ma-na 16 2/3 gin2 |
| The number 4.16.40 corresponds to the answer given in line 13. Curiously, the answer is expressed in place value notation and not, as usual, in metrological notation. |
| Col. | I | II | III | IV | |
| Reciprocal of I ×7×11 | II × 3.20 | I × III | |||
| Rate in-kind | Rate in-silver×7×11 | Value in-silver | Value in-kind (equal) | ||
| Wool 1 | 7 | 11 | 36.40 | 4.16.40 | |
| Wool 2 | 11 | 7 | 23.20 | 4.16.40 | |
| Total | 18 | 1 | |||
| Table 8: sketch of calculations for solving #12 | |||||
| #13 | The rates in-kind of two types of oil are given (7 mana per gin2 and 1 ban2 2 sila3 per gin2) that correspond to the following SVPN: |
| 7 ma-na | → 7 | |
| 1(ban2) 2 sila3 | → 12 | |
| 1 gin2 | → 1 |
| The same quantity of each type of oil is bought for the price of 1 gin2 of silver (total of column III of table 9). There is no question, but we may suppose that the reader was expected to calculate the price of each kind of oil (column III), and/or the common quantity (column IV). The rate in-kind of common oil is 7, a non-regular number, thus column II is replaced with the products of the rates in-silver by 7. The total of items of column II is 36, and the total of the items of col. III is 1. Thus, the coefficient of col. III is 1.40 (reciprocal of 36). The rest of the table is then easy to fill. The value in-kind of common oil is 11.40, of lard is 11.40, thus the total is 23.20, which corresponds to 2 ban2 3 1/3 sila3, the answer provided on the edge of the tablet (which does not appear in Neugebauer’s hand copy – see note on #13 in §5). |
| Col. | I | II | III | IV | |
| Reciprocal of I ×7 | II × 1.40 | I × III | |||
| Rate in-kind | Rate in-silver ×7 | Value in-silver | Value in-kind (equal) | ||
| Common oil | 7 | 1 | 1.40 | 11.40 | |
| Lard | 12 | 35 | 58.20 | 11.40 | |
| Total | 36 | 1 | 23.20 | ||
| Table 9: calculations for solving #13 | |||||
| #14 | Statement #14 displays similarities to N 3914 discussed in (Friberg 2007, 163-165) and to VAT 7530 #5 (obv. 17-21) discussed in (Friberg 2007, 166). The purpose of this problem is to find each quantity that corresponds to each given rate in-kind, from 1 to 9 in this problem, that will add up to a total amount paid, probably stipulated as “1(barig) še” in this problem. One of the rates in problem 14 is the number 7, a non-regular number in the sexagesimal system as in #12 and 13. Unlike the problems discussed in Friberg, problem 14 added an extra step: the scribe had to round in order to find the answer. Also, unlike both N 3914 and VAT 7530 § 5, problem 14 uses grain to evaluate the unnamed goods instead of silver, a significant deviation from the prior problems in this series. There are nine sorts of goods, for which the rates inkind are respectively 1 (unit), 2 (units), ..., 9 (units) (quantities which equivalent value in-grain is 1 sila3). The value in-kind of the goods (column IV of table 10) must be a multiple of 7, the unique non-regular rate in-kind of the problem. Thus column II is replaced with the products of the rates in-grain by 7. Following the idea of Friberg (cf. N 3914 in Friberg 2007: 163-165), we designate these products as “false value in-grain.” The coefficient providing the actual value is calculated using the information given in line 19 of the text: the total value in grain of the nine goods bought is 1 barig (corresponding to 1). The total false value in-grain is 19.48.10 (approximately 20). The total value of the purchase to be found must be 1 barig (1). The coefficient is approximately the reciprocal of 20, that is, 3. Multiplying the values of column II by 3 gives the actual values in-grain of the nine goods (column III). The value in-kind is the false quantity, 7, multiplied by the coefficient, 3, that is, 21. It is also the product of column I, the rates in-kind, by column III, the values in-grain. Since #14 exhibits some similarities with VAT 7530 obv. 17-21, this text deserves to be quoted (following Neugebauer, MKT 1, 288): |
| 1 ma-na ta-am3 2 ma-na [3 ma]-˹na˺ 4 mana, 5 mana | Per 1 mana, 2 ma-na, 3 mana, 4 ma-˹na˺ [5 ma-na] | |
| 6 ma-na 7 ma-na 8 ma-na 9 ma-na 10 ˹ma-na˺ | 6 mana, 7 mana, 8 mana, 9 mana, 10 mana, | |
| 10 gin2 igi 4-gal2 u3 ši-za-a-at[14] še ku3-babbar | 10 1/4 gin2 and 1/3 še silver | |
| ku3-babbar li-li li-ri-da-ma | Let the silver rise and fall, | |
| ganba li-im-ta-hi-ra | so that the rate in-kind is equal. |
| I | II | III | IV | ||
| (reciprocal of I × 7) | (II × 3) | (I × III) | |||
| Rate in-kind | False value in-grain | Value in-grain | Value in-kind (equal) | ||
| 1 | 7 | 21 | 21 | ||
| 2 | 3.30 | 10.30 | 21 | ||
| 3 | 2.20 | 7 | 21 | ||
| 4 | 1.45 | 5.15 | 21 | ||
| 5 | 1.24 | 4.12 | 21 | ||
| 6 | 1.10 | 3.30 | 21 | ||
| 7 | 1 | 3 | 21 | ||
| 8 | 52.30 | 2.37 30 | 21 | ||
| 9 | 46.40 | 2.20 | 21 | ||
| Total | 19.48.10 | 59.24.30 | 3.9 | ||
| Rounded | 20 | 1 | |||
| Difference | 11.50 | 35.30 | |||
| Error | 1% | ||||
| Table 10: calculations for solving #14 | |||||
| #15 | Problem 15 provides both a connection with problem 14 and a significant departure from the previous sections. It is connected to problem 14 in that it continues the use of value in-grain rather than value in-silver. However, a significant departure is seen in that the exchange rate is no longer stated as X in-kind quantity per 1 gin2 of silver, but X in-kind quantity per Y sila3 grain. The value in-kind is a number of fish. Thus we see two rates in problem 15: 3 ku6-a 5 sila3 “for 3 fish, 5 sila3,” and 5 ku6-a ku6 […] “for 5 fish ….” The exchange rate is a ratio of 3/5 (36) and 5/x respectively. These ratios can be easily converted into the same formula as in the previous problems. The data are converted in SPVN as follows: |
| 5 sila3 | → 5 | |
| 4(aš) 1(barig) 4(ban2) 5 (sila3?) | → 21.45 |
| Table 11 provides the following interpretation. Column I is the rate in-kind, 3/5 or 36. Column II is then the reciprocal, that is, 5/3 or 1.40. It is interesting to see that the goal of the problem is the same as in #14, to find the values in-grain and in-kind. However, to underline that there is a significant difference in the ratio, the -ta suffix is not employed, and the problem states in lines 5-6, ku6 še ḫe2-e3 u3 ḫe2-e11 ku6-a ib2-sa2-ma, “Let the fish and the grain rise or fall (so that) the fish are equal.” Unfortunately the second rate is broken and so we are unable to solve this problem completely. Interesting is the use of ganba to describe this problem in line 1. One would expect a different term if the use of a different form or ratio defined by both the in-kind and in-grain rate is employed. However, this use makes sense when it is understood that the problem is a rate in-kind exercise, that is, that the use of the rate in-kind must be found and employed to find the answer. If this is true, then the use of ganba as described in §2.4 is justified. This is, in the end, a rate in-kind problem and ganba’s use here informs us on how the author of this text expected to deal with an odd exchange rate. Further, we see a similar use of ganba in economic texts. As an example, we turn again to RA 15, 191 (mentioned above §2.4), this time to lines 1-3: |
| 8 gu2 siki sag-ga2 | 8 gu2 select white wool, | |
| ganba 1 gu2-e 7 1/2 | rate in-kind to 1 gu2 7 1/2 gin2, | |
| ku3-bi 1 ma-na | its value 1 mana. |
| Note that in this example the explicit lack of the -ta suffix which is present in lines 16-18 of the same document to describe another rate. The difference between RA 15, 191, and our text would be the directive/ locative -e in RA 15, 191, which is not explicitly mentioned in YBC 4698. |
| Col. | I | II | III | IV | |
| Rate in-kind | Rate in-grain | Value in-grain | Value in-kind | ||
| Fish | 1 36 (3/5) | 1.40 (5/3) | |||
| Fish 2 | ? (5/x) | ? (x/5) | |||
| Total | 21.45 | ||||
| Table 11: calculations for solving #15 | |||||
| #16 | This statement was correctly read and translated by Thureau-Dangin (1937b: 89-90; our rendering of the French):
As much as the price of lead I paid (literally weighed) some silver, I bought some lead, I added silver and lead: 7 mana. The silver and lead are what?As noted by Thureau-Dangin, the market rate of lead is missing, and the problem cannot be solved. However, it seems to us that arithmetical arguments actually demand that the rate in-kind of lead be 7. Indeed, lines 10-11 seem to indicate that the weight of lead bought and the weight of silver used for buying the lead are added and that the total weight of metal is 7 mana (total value in-kind, corresponding to 7—see table 12, col. IV). As it is stated that “As much as the rates in-kind of the lead silver I weighed and lead I bought,” the weight of lead must be equal to the weight of silver, that is, 3.30 (col. IV). Thus, the weights of both metals are 3.30. The goal of the problem is probably to find the value in silver of lead (col. III). Of course, the rate in-kind of silver is 1 and the value in-silver of silver is the same as the value in-kind of silver, that is, 3.30. For the lead, it seems that the lead’s rate is missing, as noted by Thureau-Dangin. However, we can rely on the fact that 3.30 = 7×30, thus the factor 7 appears in columns I, II and III. Therefore, the rate in-kind of lead must be 7. Since 7 is not regular, column II cannot provide reciprocals of rates in-kind, but instead provides 7 times the reciprocals of rates in-kind. The coefficient providing values in-silver (col. III) from rates in-silver is 3.30, thus the coefficient which produces col. III from col. II is 30 (because col. II is 7 time the rates in-silver). The table can be filled, and the problem can be solved. |
| 30 → 1/2 ma-na |
| The value in-silver of lead is 1/2 mana. Note the use of the verb la2 to mark the means of payment. The actor weighed out silver against the lead, and literally found the exchange ratio in the purchase. |
| Col. | I | II | III | IV | |
| Reciprocal of I × 7 | II × 30 | I × III | |||
| Rate in-kind | Rate in-silver × 7 | Value in-silver | Value in-kind | ||
| Lead | 7 | 1 | 30 | 3.30 | |
| Silver | 1 | 7 | 3.30 | 3.30 | |
| Total | 8 | 4 | 7 | ||
| Table 12: calculations for solving #16 | |||||
| #17 | Even if the situation described by this statement is not completely clear to us, it seems quite sure that: |
|
| 1) | We are dealing with two kinds of stones: common stones and gold inlaid (ku3-sig17 gar-ra) stones. 6 stones of each kind are bought. As the value of 6 common stones is 1 unit (probably 1 gin2 of silver, as usual), and the value of 6 gold inlaid stones is the same, the weight of one common stone is not the same as the weight of one gold inlaid stone. | |
| 2) | The question is probably to find the weight of each kind of stones, that is, the rate in-kind of 6 common stones and of 6 inlaid stones, as well as the corresponding rates in-silver (line 19). | |
| 3) | Lines 17 and 18 seem to describe the process of making equal the quantities of the two kinds of stones. Thus, the weight of common stone is 1/2 mana 1 1/2 gin2 (31.30), as well as the weight of inlaid stones; the total weight is 1 mana 3 gin2 (1.3), as stated in line 22. | |
| Col. | I | II | III | IV | |
| Reciprocal of I ×7 | II × 4.30 | I × III | |||
| Rate in-kind | Rate In-silver ×7 | Value in-silver | Value in-kind | ||
| (weight of stones per 1 gin) | (price of the stones) | (equal weights of stones) | |||
| Common stones | 6 a | 7 × 10 × a' | 5.15 a' | 31.30 | |
| Gold inlaid stones | 6 b | 7 × 10 × b' | 5.15 b' | 31.30 | |
| Total | 1 | 1.3 | |||
| Table 13: sketch of calculations for solving #17 | |||||
| Col. | I | II | III | IV | |
| Reciprocal of I ×7 | II × 4.30 | I × III | |||
| Rate in-kind | Rate In-silver ×7 | Value in-silver | Value in-kind | ||
| Common stones | 6 × 21 | 3.20 | 15 | 31.30 | |
| Gold inlaid stones | 6 × 7 | 10 | 45 | 31.30 | |
| Total | 1 | 1.3 | |||
| Table 14: possible sketch of calculations for solving #17 | |||||
| Now, if we observe that 31.30 is 7×4.30, we guess that the factor 7 appears in rates in-kind, and that the situation is similar to #12. Thus, column II of Table 13 contains the products of reciprocals of column I by 7 (in the table, a is the weight of one common stone, and a' the reciprocal of a; b is the weight of one inlaid stone, and b' the reciprocal of b). We can now calculate the factor k providing column III from column II. Indeed, since IV is I × III, we have: |
| 31.30 | = 6 a × 7 × 10 × a' × k |
| Thus |
| k = | 4.30 |
| The values of a and b can be calculated, assuming that the total of column III is 1, as usual: |
| 5.15 (a' + b') | = 1 | |
| 7 × 45 (1/a + 1/b) | = 1 | |
| (a + b)/ab | = 1/7 × 1.20 |
| This means that a or b is a multiple of 7. Of course, an infinity of solutions is possible. Say, for simplicity, that b = 7; thus a = 21. The starting point of this analysis of the problem is the answer (the equal weights of the two kinds of stones is 1/2 mana 1 1/2 gin2), that is, we filled in Table 13 from col. IV to col. I. Some data seem missing in order to fill the table from col. I to col. IV. However, suppose that it was already stated in a previous tablet of this series that inlaid stone has three times more value than common stone: if the weight of one inlaid stone is n, then, the weight of one common stone is 3n. Moreover, suppose that, in order to solve the problem, the column II provides the false value equal to “Reciprocal of I ×n.” In this way the problem can be solved. A crucial test, to evaluate this hypothesis, should be to decipher the first signs of line 21, which remain unclear for us. |
§7. Conclusion
§7.1. Some of the problems of the text remain unclear or uncertain. However, the improvement of the understanding of the text we tried to offer, compared to Neugebauer, Thureau-Dangin and Friberg’s previous publications, lies in the meaning of the whole text. This set of 17 problems presents a strong consistency, and most of the statements appear as variations of a basic mathematical sketch. We tried to underline this single mathematical framework by referring systematically to the same chart, represented by conversions and a table of data, which emerges from school exercises (§3.2 and §3.3). In diverse situations, direct and reverse problems are built by suggesting different paths in filling the corresponding table of data.
§7.2. Some of the uncertainties of interpretation originate in our lack of pieces of information. This probably results from the fact that this tablet is just one tablet in a series (the third one), and that relevant information may have been provided in the first two tablets of the series. This detail underlines the importance of considering texts as wholes, when possible. It must be noted that some of the statements have parallels in the known corpus of mathematical procedure texts and school exercises. This means that the author of the tablet reused old mathematical material. But, unlike catalogue texts, the list of statements noted on YBC 4698 doesn’t seem to be a compilation gathering statements from different sources, but rather a systematic elaboration of new material from old material. This is a typical feature of series texts (Proust 2009b).
§7.3. Another striking feature of this text is the way in which Akkadian expressions used elsewhere in mathematical texts are translated word for word into a quite artificial Sumerian language. The trace of Akkadian expressions is particularly clear in problems 14 and 15 (še ḫe2-e3 u3 ḫe2-e11-ma ganba ib2-sa2). The use of Sumerograms such as bur2, e3 and e11 seems to be specific to this text, since only the Akkadian counterparts are attested in other mathematical texts. These linguistic features probably reflect the habits of a highly erudite milieu.
§8. Indices
§8.1. Glossary
| a-na | as much as |
| an-bar | iron |
| bur2 | to sell |
| DUG | object (unclear meaning) |
| e3 | to rise |
| e11 | to fall |
| en-nam | how much? |
| ganba | rate (in-kind or in-silver) |
| gar-gar | to add |
| i3-geš | common oil |
| i3 sag | first quality oil |
| i3-šah2 | lard |
| ib2-sa2 | to make equal |
| ki-la2 | weight, to weigh |
| ku3-babbar | silver |
| ku3-babbar diri | silver profit |
| ku3 diri | silver profit (abbreviation) |
| ku3-sig17 | gold |
| ku3-sig17 gar-ra | inlaid gold |
| ku3-sum | to pay |
| ku3 la2 | to weigh silver, to pay |
| ku6 | fish |
| la2 | to weigh |
| maš2 | interest |
| na4 | stone |
| nagga | lead |
| sa10 | v. to buy, to make equivalent; n. purchase, equivalent |
| siki | wool |
| šum2 | to give |
| šu-ti-a | received |
| šuš4 | to cut |
| zu | to know |
§8.2. Metrological systems
Units of capacities (1 sila3 ≈ 1 liter)
| gur | ←×5← | barig | ←×6← | ban2 | ←×10← | sila3 |
| sila3 | ←×60← | gin2 | ←×180← | še |
Units of weight (1 ma-na ≈ 500 g)
| gu2 | ←×60← | ma-na | ←×60← | gin2 | ←×180← | še |
§8.3. Metrological tables (outline)
Capacities
| 1 še | 20 |
| 1 gin2 | 1 |
| 1 sila3 | 1 |
| 1(ban2) | 10 |
| 1(barig) | 1 |
| 1 gur | 5 |
Weights
| 1 še | 20 |
| 1 gin2 | 1 |
| 1 ma-na | 1 |
| 1 gu2 | 1 |
The complete OB metrological tables according to Nippur sources can be found in Proust 2009a: §8.
§9. Neugebauer archives
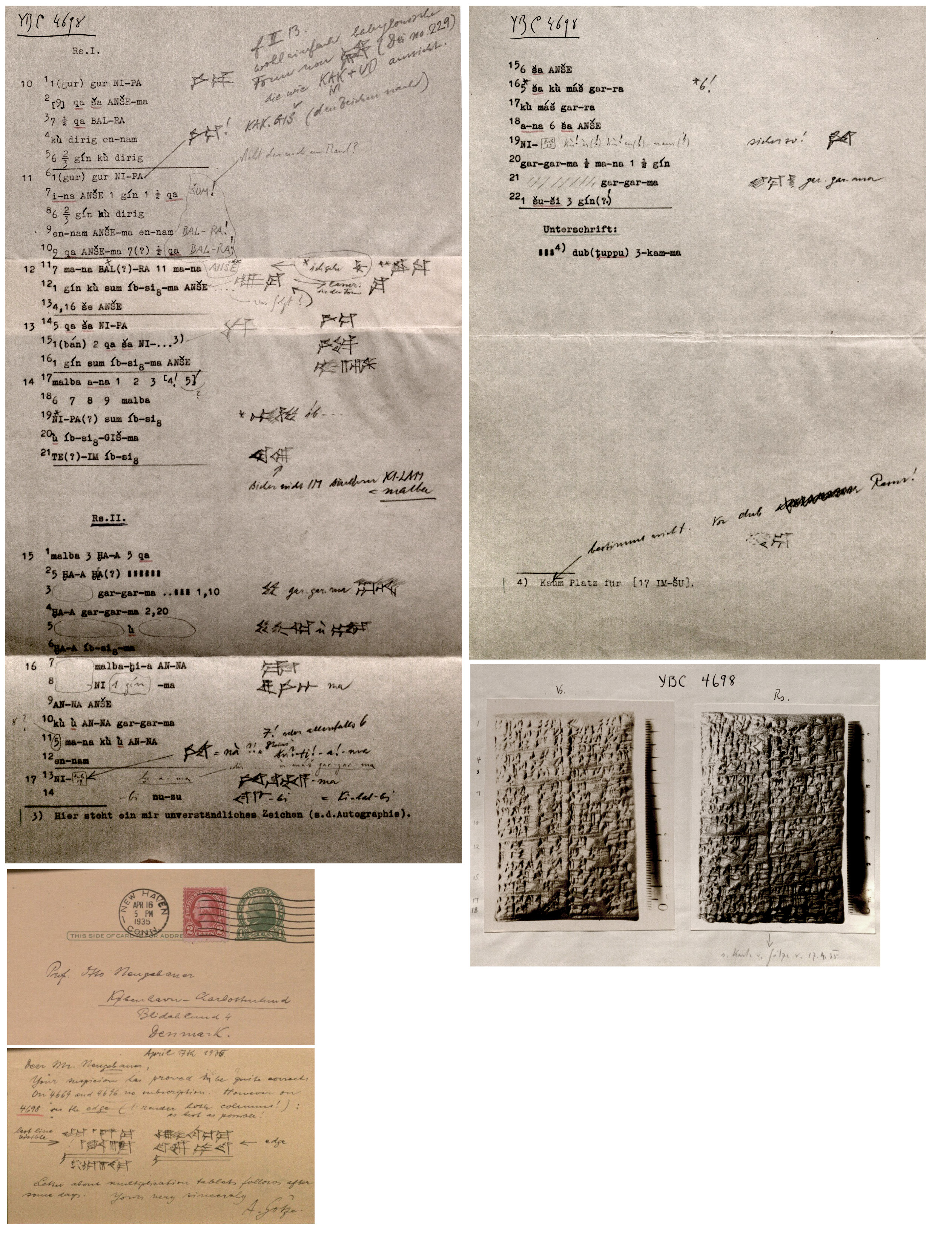
A copy of the folder “YBC 4698” from the Aaboe-Britton Archives is provided in figure 1. These archives, which contain the documents used by Neugebauer for the publication of MKT and MCT, are kept at the Institute for the Study of the Ancient World, New York University. We thank Alexander Jones for scanning these documents for us and allowing us to present them here.
The folder contains
1) the transliteration of the reverse of YBC 4698 by Neugebauer, with annotations by Albrecht Goetze
2) a postcard sent by Goetze to Neugebauer on April 17, 1935
3) the photographs used by Neugebauer to publish the text in MKT 3, 42-45
These photographs are not included in MKT. Thureau-Dangin used copies of the same photographic negative, sent to him by the curator, Ferris J. Stephens. More detail on Aaboe-Britton Archives and on the way in which Neugebauer and Thureau-Dangin worked with photographs can be found in (Proust & Rougemont forthcoming).
Figure 1: Aaboe-Britton Archives, folder “YBC 4698” (courtesy of ISAW/NYU)
Notes
|
1 The research leading to this article has received funding from the European Research Council under the European Union’s Seventh Framework Program (FP/2007-2013) / SAW Project led by Karine Chemla. This article is the result of discussions among the members of SAW project developed during the academic year 2011-2012. All of the participants have to be warmly thanked for their contributions, especially Bertrand Lafont and Cécile Michel, who spent time in reading the first drafts and improving the final version. Many of the new readings are due to Antoine Cavigneaux, to whom we are heavily indebted. We particularly thank Jöran Friberg for his many corrections and suggestions, and the anonymous CDLJ reviewers for their constructive critiques. Remaining errors or omissions are the lone responsibility of the authors. This work is based on the examination of the tablet at Yale made by the authors in 2009 and 2012. We thank Benjamin Foster and Ulla Kasten for their collegial reception, and their authorization to work on the tablet. 2 “The text bears the single line colophon x x ṭuppu 3-kamma. The number of subjects (or examples) is 17. These are grain (še), silver (kù-babbar), interest (máš) and the like, but in detail there are still so many difficulties that I do not wish to give a full transcription” (MKT 1, 513 [tranlsation of the German by the authors]). 3 “Several points in this text remain unclear to me. Aside from the difficult readability, this is due above all to the fact that obvious conventions and terms of the economy to be taken as a given are not known (at least to me)” (MKT 3, 43 [tranlsation of the German by the authors]). 4 The tablet is mentioned in Legrain 1937: 1947 in connection with UET 3, 1377. See also Thureau-Dangin 1937a: 80-86); Bruins & Rutten 1961 in connection with TMS 13; Nemet-Nejat 1993: 57, 60, 61-63, 86, with some comments. 5 VAT 7530 is published in MKT 1, 287-289, and in TMB 100; MLC 1842 is published in MCT 106-107. See also the list of problems dealing with compound interests in Nemet-Nejat 1993: 58-61, AO 6770 #2, VAT 8521, VAT 8528, YBC 4669 #11. 6 TMS 13 and 22 are published in Bruins and Rutten 1961: 82-83, 111-114. 7 Baqir 1951; see also the bibliography provided by Gonçalves forthcoming: 68. 8 Friberg & George 2010: 149-154. 9 See MKT 3, 74: pašārum = “verkaufen” [to sell]. In MCT 107, note 276h states “As pointed out by Waschow [2] p. 246a, the verb pšr occurs in two badly preserved mathematical texts (VAT 6469 and VAT 6546, both published MKT 1, p. 269) dealing with purchases. Waschow translates pšr by “einlösen, für Geld weggeben” [to redeem, to give away for money]. In translating this same text, Friberg equates bur2 with “to sell” (2005: 216). 10 See TMB 221 under “maḫiru,” MCT 106 and note 276e. Particularly interesting is the example cited by Neugebauer and Sachs in note 276e: “In the case of HE 113 (published by Scheil, RA 15, 184-185; republished by Boyer CH pl. 6 and pp. 33ff.), line 6, the phrase ganba a-na 1 gin2 heads a column containing entries which give the quantity of fish of various sorts corresponding to 1 gin2 of silver.” We come back in §3 on the use of tabular presentation for rates in-kind and in-silver in mathematical texts. 11 “Those texts of Yale that make up series employ a term frequently written a-na where N. [Neugebauer] sees the Akkadian preposition ana “to,” “for,” etc.. This preposition is never supposed “to govern” anything, for which N. does not seem surprised. In reality, a-na is here the ideogram mala.” (Thureau-Dangin 1936: 57 [translation of the French by the authors]). 12 See also Nemet-Nejat 1995: 256 and Proust 2007: 202-205 for other examples of similar texts. 13 Note that the inscription on the bottom edge appears to be above the table, which is on the reverse. Indeed, the tablets are usually turned around the bottom edge. The obverse of the tablet is a list of statements of five problems dealing with sharing an amount of silver between four brothers. Friberg doesn’t establish a relationship between the obverse and the reverse. 14 Note that Friberg (2007: 166) reads in line 19 “igi 4-a-at,” translated “1/4th part,” against Neugebauer’s “ši-za-at,” translated “ein Sechstel (??).” Friberg argues convincingly that “the solution to the problem VAT 7530 §3 is given in the form of a tabular array, where the number 1 03 45 corresponds to 1 gin2 11 še igi 4-a-at še” (Friberg, personal communication); see also Friberg 2007: 167. |
Bibliography
| Abbreviations | ||
| MCT = Neugebauer and Sachs 1945 | ||
| MKT 1 = Neugebauer 1935 | ||
| MKT 3 = Neugebauer 1937 | ||
| TMB = Thureau-Dangin 1938 |
| Bruins, Evert M. & Rutten, Marguerite M. | ||
| 1961 | Textes mathématiques de Suse. Paris: Geuthner | |
| Friberg, Jöran | ||
| 2005 | Unexpected Links Between Egyptian and Babylonian Mathematics. Singapor: World Scientific | |
| Publishing Co. | ||
| 2007 | A Remarkable Collection of Babylonian Mathematical Texts, vol. I. New York: Springer | |
| 2011 | Review of Proust, Tablettes Mathématiques de Nippur, 2007. Archiv für Orientforschung, 52, 259-263 | |
| Friberg, Jöran & George, Andrew R. | ||
| 2010 | “Six More Mathematical Cuneiform Texts in the Sch.yen Collection.” In D. Minutoli & R. Pintaudi, eds., Papyri Graecae Schøyen. Essays and Texts in Honour of Martin Schøyen. Florence: Edizioni Gonnelli, pp. 123-195 | |
| Gonçalves, Carlos | ||
| forthcoming | A Dozen Mathematical Tablets from Tell Harmal. Berlin, London: Springer | |
| Gundlach, Karl-Bernhard & von Soden, Wolfram | ||
| 1963 | “Einige altbabylonische Texte zur L.sung ‘quadratischer Gleichungen’.” Abhandlungen aus dem mathematischen Seminar der Universität Hamburg 26, 248-263 | |
| Høyrup, Jens | ||
| 2002 | Lengths, Widths, Surfaces. A Portrait of Old Babylonian Algebra and its Kin, Studies and Sources in the History of Mathematics and Physical Sciences. Berlin, London: Springer | |
| Jacobsen, Thorkild | ||
| 1946 | Review of Mathematical Cuneiform Texts in BASOR 102, 17-18 | |
| Legrain, Leon | ||
| 1937, 1947 | Business documents of the Third Dynasty of Ur, vol. 3. London, Philadelphia: British Museum / University of Pennsylvania | |
| Nemet-Nejat, Karen R. | ||
| 1993 | Cuneiform Mathematical Texts as a Reflection of Every Day Life in Mesopotamia. AOS 75. New Haven: American Oriental Society | |
| 1995 | “Systems for Learning Mathematics in Mesopotamian Scribal Schools.” JNES 54, 241-260 | |
| 2002 | Square Tablets in the Yale Babylonian Collection. In J. M. Steele & A. Imhausen, eds., Under One Sky: Astronomy and Mathematics in the Ancient Near East. AOAT 297. Münster: Ugarit-Verlag, pp. 253-281 | |
| Neugebauer, Otto | ||
| 1935 | Mathematische Keilschrifttexte I. Berlin: Springer | |
| 1937 | Mathematische Keilschrifttexte II-III. Berlin: Springer | |
| Neugebauer, Otto & Sachs, Abraham | ||
| 1945 | Mathematical Cuneiform Texts. AOS 29. New Haven: American Oriental Society | |
| Proust, Christine | ||
| 2007 | Tablettes mathématiques de Nippur. Varia Anatolica 18. Istanbul: IFEA, De Boccard | |
| 2009a | “Numerical and Metrological Graphemes: From Cuneiform to Transliteration.” CDLJ 2009:1 | |
| 2009b | “Deux nouvelles tablettes math.matiques du Louvre: AO 9071 et AO 9072.” ZA 99, 167-232 | |
| forthcoming | “Mathematical and Philological Insights on Cuneiform Texts. Neugebauer’s Correspondence with Fellow Assyriologists.” In A. Jones, C. Proust & J. Steele, eds., A Mathematician’s Journeys. Otto Neugebauer Between History and Practice of the Exact Sciences | |
| Snell, Daniel C. | ||
| 1982 | Ledgers and Prices: Early Mesopotamian Merchant Accounts. YNER 8. New Haven: Yale University Press | |
| Steinkeller, Piotr | ||
| 1989 | Sale Documents of the Ur III Period. FAOS 17. Stuttgart: Franz Steiner | |
| Thureau-Dangin, Fran.ois | ||
| 1936 | “Compte rendu de MKT I (1935).” RA 33, 55-62 | |
| 1937a | “La mesure du “qa”.” RA 34, 80-86 | |
| 1937b | “Bibliographie: O. Neugebauer, MKT III.” RA 34, 87-92 | |
| 1938 | Textes Mathématiques Babyloniens. Leiden: Ex Oriente Lux | |
| Waschow, Heinz | ||
| 1936-1937 | “Review of Neugebauer, MKT I-II.” AfO 11, 244-247 | |
| 1939 | “Review of Neugebauer, MKT III.” AfO 12, 277 | |
Version: 13 June 2014